Derivative of sin3x: Proof by First Principle, Chain Rule

The derivative of sin 3x is cos 3x. In this article, we will find the derivative of sin 3x by the substitution method, the chain rule, and the limit definition.

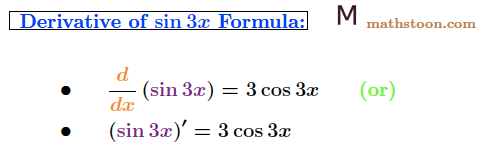
Derivative of sin 3x Formula

The formula of the derivative of sin 3x is given below.

• d/dx(sin 3x) = 3cos 3x  (or)
• (sin 3x)$’$ = 3 cos 3x

The following method will be used to prove the above formula.

• Derivative of sin 3x by substitution method
• Derivative of sin 3x by first principle
• Derivative of sin 3x by chain rule.

What is the Derivative of sin 3x?

At first, we will evaluate the derivative of sin 3x by the substitution method. We need to follow the below steps.

Step 1: Let $y=\sin 3x$

Step 2: Applying sine inverse on both sides, we have

$\sin^{-1} y =\sin^{-1} \sin 3x$

$\Rightarrow \sin^{-1} y =3x$

Step 3: Differentiating with respect to $x$, we get

$\dfrac{d}{dx}(\sin^{-1}y)=\dfrac{d}{dx}(3x)$

$\dfrac{1}{\sqrt{1-y^2}} \dfrac{dy}{dx}=\dfrac{d}{dx}(3x)$

$\Rightarrow \dfrac{1}{\sqrt{1-y^2}} \dfrac{dy}{dx}=3$

$\Rightarrow \dfrac{dy}{dx}=3\sqrt{1-y^2}$

$\Rightarrow \dfrac{dy}{dx}=3\sqrt{1-\sin^2 3x}$ $[\because y=\sin 3x]$

$\Rightarrow \dfrac{dy}{dx}=3\sqrt{\cos^2 3x}$ $[\because \sin^2 3x+\cos^2 3x=1 ]$

$\Rightarrow \dfrac{dy}{dx}=3\cos 3x$

So the derivative of sine 3x is $3\cos 3x$. That is,

\[\dfrac{d}{dx}(\sin 3x)=3\cos 3x. \quad ♣\]

Video Solution of derivative of sin 3x:

Derivative of sin 3x by first principle

Now, we will find the derivative of $\sin 3x$ by the first principle. Let $f(x)=\sin 3x.$ Applying the first principle of derivatives, we get that

$\dfrac{d}{dx}(f(x))$ $=\lim\limits_{h \to 0}\dfrac{f(x+h)-f(x)}{h}$

Thus the derivative of sin 3x by limit definition is equal to

$\dfrac{d}{dx}(\sin 3x)$ $= \lim\limits_{h \to 0} \dfrac{\sin 3(x+h)-\sin 3x}{h}$

$=\lim\limits_{h \to 0}\dfrac{2\cos {\frac{6x+3h}{2}} \sin {\frac{3h}{2}}}{h}$

$[\because \sin a -\sin b$ $=2\cos \frac{a+b}{2}\sin \frac{a-b}{2}]$

$=\lim\limits_{h \to 0}\cos \big(\dfrac{6x+3h}{2}\big)\cdot$ $3\cdot \dfrac{\sin \frac{3h}{2}}{3h/2}$

$=3\lim\limits_{h \to 0}\cos \big(\dfrac{6x+3h}{2}\big)$ $\lim\limits_{h \to 0}\dfrac{\sin \frac{3h}{2}}{3h/2}$

[Let 3h/2=t. Then t → 0 as h → 0]

$=3\cos \big(\dfrac{6x+0}{2}\big) \lim\limits_{t \to 0}\dfrac{\sin t}{t}$

$=3\cos 3x \cdot 1$

$=3\cos 3x$

This shows that the derivative of sin 3x is 3cos 3x which is obtained by the first principle of derivatives, that is, by the limit definition. ♣

Also Read: 

Derivative of root x: The derivative of √x is 1/2√x

Derivative of cube root of x: The derivative of the cube root of x is 1/(3x^{2/3})

Derivative of sin inverse x: The derivative of  sin^-1 x is 1/√(1-x²)

Integration of mod x: The integration of  |x| is -x|x|/2 +c

Derivative of Sin 3x by chain rule

We know that the derivative of sin x is cos x, that is, $\frac{d}{dx}(\sin x)=\cos x.$ Using this formula and by the chain rule, we will now find the derivative of sine 3x.

Recall the chain rule of derivative: $\frac{du}{dx}=\frac{du}{dz} \cdot \frac{dz}{dx},$ where $u$ is a function of $z$. To find the derivative of $\sin 3x$ by the chain rule, we put

$z=3x$

Now, $\dfrac{d}{dx}(\sin 3x)=\dfrac{d}{dx}(\sin z)$

$=\dfrac{d}{dz}(\sin z) \cdot \dfrac{dz}{dx}$ (by the chain rule)

$=\cos z \cdot 3$

$=3\cos z$

$=3\cos 3x$ $[\because z=3x]$

So the derivative of sin3x by the chain rule is equal to 3cos3x.

FAQs on Derivative of Sin 3x

Back to HOME PAGE