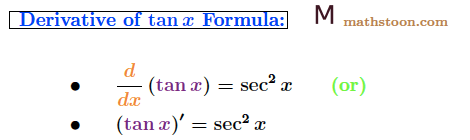
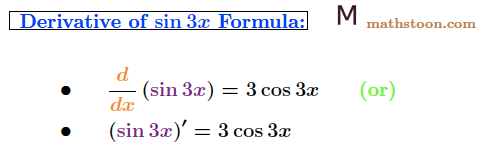
Derivative of e^cosx: Formula, Proof by First Principle, Chain Rule
Differentiate e^cos x. The derivative of ecos x is -sin x ecos x. In this post, we will calculate the derivative of e to the power cos x by the first principle of derivatives as well as by the logarithmic differentiation and the chain rule of derivatives. We will also solve a few questions related … Read more